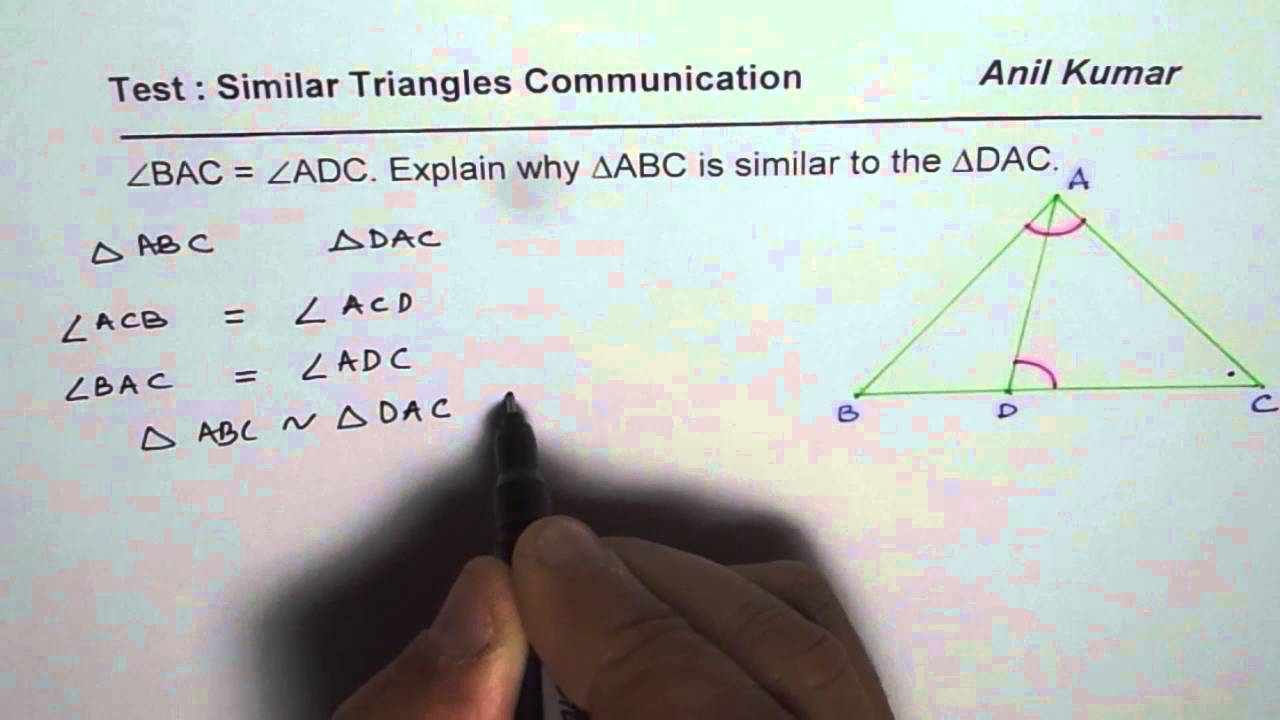
200以上 one way to show that two triangles are similar is to show that 277413-One way to show that two triangles are similar is to show that
May 05, 19 · In the diagram of similar triangles the corresponding sides are the same color Naming similar triangles To show two triangles are similar, you use the symbol ???\sim???Two triangles are similar if they share all three angles Since the angles of a triangle sum to 180 degrees, they only need to share two angles in order for you to figure out they're similar Similar triangles are useful since if triangle ABC is similar to triangle DEF, A/D = B/E = C/F, and the same is true for the angles 225 viewsIf two objects have the same shape, they are called "similar" When two figures are similar, the ratios of the lengths of their corresponding sides are equal To determine if the triangles shown are similar, compare their corresponding sides Are these ratios equal?
Congruent Triangles On The Coordinate Plane
One way to show that two triangles are similar is to show that
One way to show that two triangles are similar is to show that-View 7jpg from MATH Algebra II at Flower Mound H S 1 One way to show that two triangles are similar is to show that 65 1 1 a two angles of one are congruent to two angles of the other b twoAll of these methods can be used to show that two triangles are similar EXCEPT A) SS B) SAS C) AA D) SSS


What Is The Congruence Of Triangles Quora
The triangles are congruent if, in addition to this, their corresponding sides are of equal length The side lengths of two similar triangles are proportional That is, if Δ U V W is similar to Δ X Y Z , then the following equation holdsSo, the two angles marked in blue are also equal With two pairs of matching angles, the two triangles are similar Memorize the appearance of this diagram, and if you spot it on the GRE, go straight to finding the ratio between side lengths The second diagram shows a triangle drawn within another triangle The two bases are parallel👉 Learn how to solve for the unknown in a triangle divided internally such that the division is parallel to one of the sides of the triangle The triangle p
Jan 21, · As we saw with the AA similarity postulate, it's not necessary for us to check every single angle and side in order to tell if two triangles are similar Thanks to the triangle sum theorem, all we have to show is that two angles of one triangle are congruent to two angles of another triangle to show similar trianglesThe Third Angle Theorem states that if two angles in one triangle are congruent to two angles in another triangle, the third angle must be congruent also The AA Similarity Postulate If two angles in one triangle are congruent to two angles in another triangle, then the triangles are similarAnswer show that the three sets of corresponding sides are in proportion If the three sets of corresponding sides of two triangles are in proportion, the triangles are similar
Theorem for Areas of Similar Triangles It states that "The ratio of the areas of two similar triangles is equal to the square of the ratio of any pair of their corresponding sides" Consider the following figure, which shows two similar triangles, \(\Delta ABC\) and \(\Delta DEF\) Theorem for Areas of Similar Triangles tells us thatSo, similar triangles are proportional to one another Just because two triangles look similar does not mean they are similar triangles in the mathematical sense of the word Checking that the corresponding angles have equal measure is one way of being sure the triangles are similarLet's review the task of proving that two triangles are similar Recall that congruence means that the measures of all three sides and all three angles are the same for the two triangles For similarity, all that is required is that the angles be congruent Another way of looking at congruence and similarity is by way of geometric transformations



Proving Similar Triangles Learning Task



If Abc And Def Are Two Triangles Such That Ab Are Respectively Equal And Parallel To De Efthen Brainly In
Note There are many ways to show that two triangles are congruent This tutorial shows you how to use a triangle congruence postulate to show that two triangles on the coordinate plane are congruent to each other!128 Chapter 3 Angles and Triangles 34 Lesson Lesson Tutorials Key Vocabulary indirect measurement, p 129 Angles of Similar Triangles Words When two angles in one triangle are congruent to two angles in another triangle, the third angles are also congruent and the triangles are similarNow show that triangles ABC and ACE are similar As before, it follows from the AA postulate that these two triangles are similar Angle A is congruent to angle A and angle C is congruent to angle E Thus, r/b=b/c Multiplying both sides by bc we get rc=b^2 Now when we add the two results we get sc rc = a^2 b^2 c(sr) = a^2 b^2 c^2 = a



Sas Similarity Read Geometry Ck 12 Foundation


Math Scene Triangles Lesson 1
(MATH!) The two triangles above are similar a Find x using the ratio of the sides 12 cm and 16 cm x/ = 12/16 Show your work b Find x using the ratio of the sides 6 cm and 8 cm Show your work c ExplainSketch and label a diagram similar to the one shown Include triangles showing the locations of the objects and their shadows 4 Explain why the two triangles are similar 5 Calculate the height of the fl agpole Show your work Solve Problems Using 14 Similar Triangles 30 MHR • Chapter 1Triangle ABC is similar to triangle


Solved One Way To Show That Two Triangles Are Similar Is Chegg Com



Similar Triangles Solutions Examples Videos
Feb 07, 12 · Favorite Answer A) If two angles of one triangle are congruent to two angles of the other triangle, it means even the third one is congruent, which implies both triangles are similarMay 07, 18 · Here are the results of two of the dilations in that activity involving triangles One of the things I really enjoy about the curriculum is the foreshadowing of the big ideas in a unit In the case of Unit 2, these images show how students work with similar triangles in Lesson 4 in a way that will pay dividends in Lesson 10 where slope isYou need to match the letters from the first triangle to the angles with the corresponding vertices on the second triangle



Ixl Sss Sas Asa And s Theorems Geometry Practice



Similar Triangles How To Prove Definition Theorems Video
If two angles of one triangle are congruent to two angles of another triangle, then they are similar Work with the class to fill in any missing angles Encourage them to use their miniwhiteboards as they workD Line k is parallel to line l mc0011jpg Which angle is congruent to mc0012jpg?,Sal uses a clever proof involving similar triangles to show that slope is constant for a line Sal uses a clever proof involving similar triangles to show that slope is constant for a line If you're seeing this message, it means we're having trouble loading external resources on our website



7 3 Proving Triangles Similar Ways To Show


Triangle Similarity Sss Sas a Postulates Proving Similar Triangles Two Column Proofs Youtube
If two angles of one triangle are respectively equal to two angles of another triangle, then the two triangles are similar, because the third angle in both triangles must be equal Show that ΔPST ~ ΔPQR The perimeters of two similar triangles ABC respectively 36 cm and 24 cm If PQ = 10 cm, find AB SolutionHow do we show that two triangles are similar based on their angles alone?Figure 2 Perimeter of similar triangles Figure 3 shows two similar right triangles whose scale factor is 2 3 Because GH ⊥ GI and JK ⊥ JL , they can be considered base and height for each triangle You can now find the area of each triangle Figure 3 Finding the areas of similar right triangles whose scale factor is 2 3



Similar Triangles



Proving The Triangle Midsegment Theorem Video Lesson Transcript Study Com
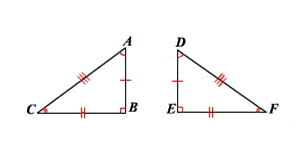
SAS similarity If two triangles have two pairs of sides that are proportional and the included angles are congruent, then the triangles are similar Measuring with Shadows Using shadows is a quick way to estimate the heights of trees, flagpoles, buildings, and other tall objectsOne way to show that two triangles are similar is to show that ______Their corresponding sides are proportional Example 121


The Easy Guide To The 30 60 90 Triangle



In The Given Figure Abc 75 Edc 75 State Which Two Triangles Are Similar And By Which Test Also Write The Similarity Of These Two Triangles By A Proper One
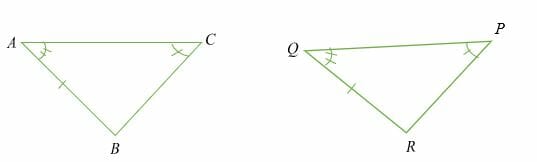
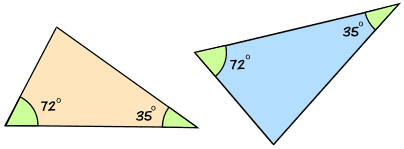
If we can show that two angles of one triangle are congruent to the two angles of the other triangle Then, obviously the third angle of first triangle will be congruent to the third angle of the second triangle Then, by the AAA property of similar triangles, we will get that both triangles are similar Hence, option (a) is correctBy the SideAngleSide Similarity Theorem (SASS), if two sides of a triangle are in proportion with the corresponding sides of another triangle, and the included angles are congruent, then the triangles are similar and, so by the Division Property of Equality, Also, and, their respective included angles, are both right angles, soExample these two triangles are similar If two of their angles are equal, then the third angle must also be equal, because angles of a triangle always add to make 180° In this case the missing angle is 180° − (72° 35°) = 73°



Similar Triangles How To Prove Definition Theorems Video


Similar Triangles Congruency Two Shapes Are Congruent If
👉 Learn how to solve with similar triangles Two triangles are said to be similar if the corresponding angles are congruent (equal) Note that two triangles2) AA similarity If two angles of one triangle are respectively equal to tow angles of another triangle, then the two triangles are similar Example In ΔPQR and ΔDEF, ∠P = ∠D, ∠R = ∠F then ΔPQR ~ ΔDEF by AA criteria 3) SSS similarity If the corresponding sides of two triangles are proportional, then the two triangles are similarJul 11, 13 · Similar Triangles Similar Triangles are the exact Same Shape, but are Different Sizes In the remainder of this lesson we will be looking at Similar Triangles The following example of two similar triangles involves one triangle, and then a second half size copy of the triangle For any two similar triangles their angles will be identical



6 4 Prove Triangles Similar By



Can You Prove That The Two Triangles Are Similar Justify Your Answer Brainly Com
For instance if the similarity ratio of 2 triangles is $$\frac 3 4 $$ , then their areas have a ratio of $$\frac {3^2}{ 4^2} = \frac {9}{16} $$ Let's look at the two similar triangles below to see this rule in action Example 1 It's easiest to see that this is true if you look at some specific examples of real similar trianglesThe graph shows a line and two similar triangles mc0191jpg What is the equation of the line?In this first problem over here we're asked to find out the length of this segment segment seee and we have these two parallel lines a B is parallel to de and then we have these two essentially transversals that form these two triangles so let's see what we can do here so the first thing that might jump out at you is that this angle and this angle are vertical angles so they are going to be


How To Prove That Two Triangles Are Similar Youtube



Similar Triangles How To Prove Definition Theorems Video
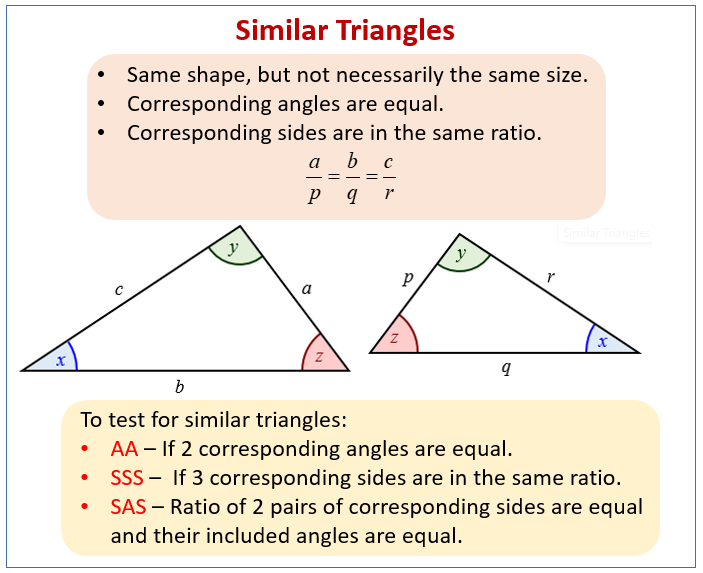
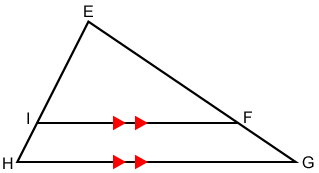
Dilations that shows this Be specific in your descriptions 4) The diagram has a pair of figures, one larger than the other For the pair, show that the two figures are similar by identifying a sequence of translations, rotations, reflections, and dilations that takes the smaller figure to the larger one 5) These two triangles are similarTo decide whether two triangles are similar, it turns out that we need to verify only one of the two conditions for similarity, and the other condition will be true automatically Similar Triangles Two triangles are similar if either their corresponding angles are equal, or;For example, triangle DEF is similar to triangle ABC as their three angles are equal The length of each side in triangle DEF is multiplied by the same number, 3, to give the sides of triangle ABC In general If two triangles are similar, then the corresponding sides are


Using Similar Triangles Examples Solutions Videos Lessons Worksheets Games Activities


Congruent Triangles Explanation Examples
May 05, 11 · Two triangles can be proved similar by the angleangle theorem which states if two triangles have two congruent angles, then those triangles are similar This theorem is also called the angleangleangle (AAA) theorem because if two angles of the triangle are congruent, the third angle must also be congruentLikewise if the measures of two sides in one triangle are proportional to the corresponding sides in another triangle and the including angles are congruent then the triangles are similar $$\frac{AB}{DE}=\frac{BC}{EF}=\frac{AC}{DF}$$ If a line is drawn in a triangle so that it is parallel to one of the sides and it intersects the other twoOne way to show that two triangles are similar is to show that _____ two angles of one are congruent to two angles of the other (AA) congruent If two polygons are similar, then the ratio of the lengths of two corresponding sides is called the _____ scale factor The _____ or two similar figures will use the same scale factor as side ratios



Consider The Two Triangles To Prove That The Triangles Are Similar By The Sas Similarity Theorem It Brainly Com



Prove That If In Two Triangles A Pair Of Corresponding Sides Is Proportional And The Including Angles Are Equal Maths Triangles Meritnation Com
Example 2 Determine the ratio of the areas of the two similar triangles Example 3 If the area of the smaller triangle is m 2, determine the area of the bigger triangle Show Stepbystep Solutions Application of Similar Triangles Example An abstract artist wants to create two proportional triangular paintingsApr 10, · Congruent Similar Neither Two sidesideside triangles are shown Each side of one triangle is equivalent to the TRIANGLE HELP!!!!Test one simply states that if two triangles are similar they must be an equiangular Both these triangles have the same shape but one is bigger than the other However, they both have the same sized angles So, we say they are equiangular


Pythagorean Theorem Wikipedia


Math Scene Triangles Lesson 1
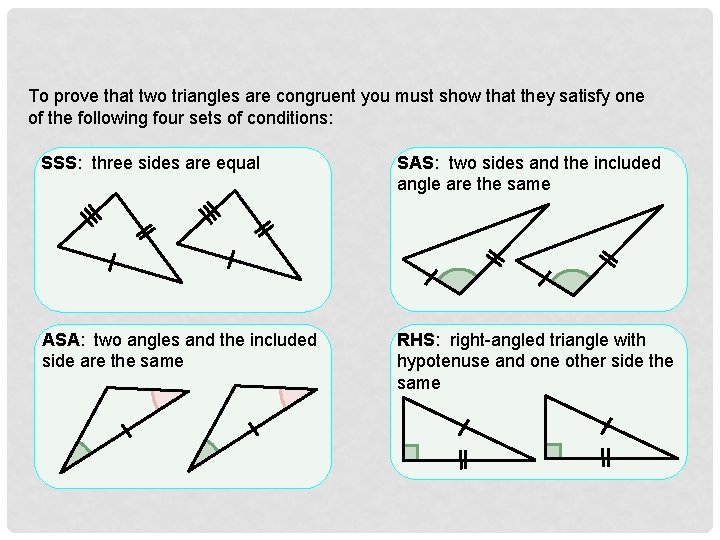
Doing so, in some cases, allows us to acquire additional information for our analysis In the case of triangles, we can apply various techniques to show that two triangles are the same or that they are similar If two triangles have sides of the same length and angles of the same measure, then we say that they are congruent triangles


Similar Triangles



Congruence And Similarity Lesson Article Khan Academy



Congruent Triangles On The Coordinate Plane



Grade 8 Mathematics Unit 2 6 Open Up Resources
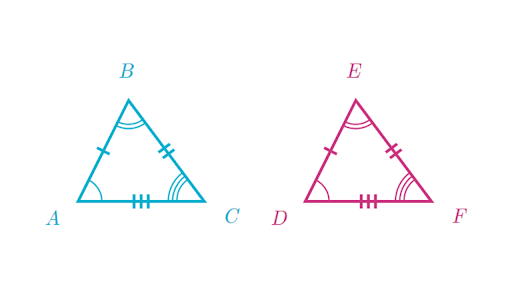


Congruence And Similarity Lesson Article Khan Academy


Congruence Geometry Wikipedia


Is a Angle Angle Angle A Congruence Rule Expii


Triangles



Similar Triangles How To Prove Definition Theorems Video



Solved One Way To Show That Two Triangles Are Similar Is To Show That A Two Angles Of One Are Congruent To Two Angles Of The Other B Two Sides O


What Is The Congruence Of Triangles Quora
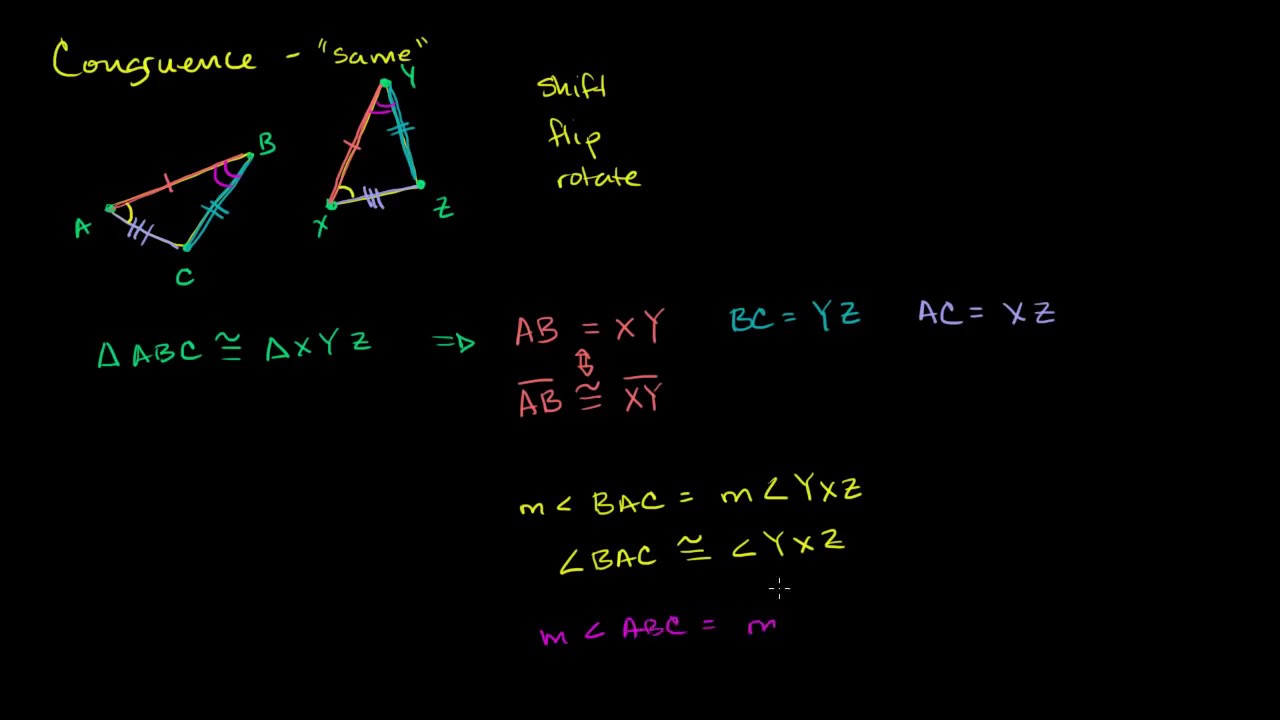


Corresponding Parts Of Congruent Triangles Are Congruent Video Khan Academy


Similar Triangles Solutions Examples Videos



Congruence Geometry Wikipedia



Congruent Triangles Criteria Geogebra



Similar Triangle Criteria Ppt Download


Tangent Ratio Read Trigonometry Ck 12 Foundation



Congruence Geometry Wikipedia



Similar Triangles


Proving Similar Triangles Mathbitsnotebook Geo Ccss Math



Grade 8 Mathematics Unit 2 6 Open Up Resources
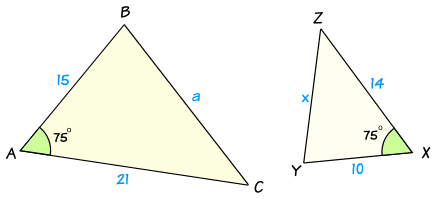


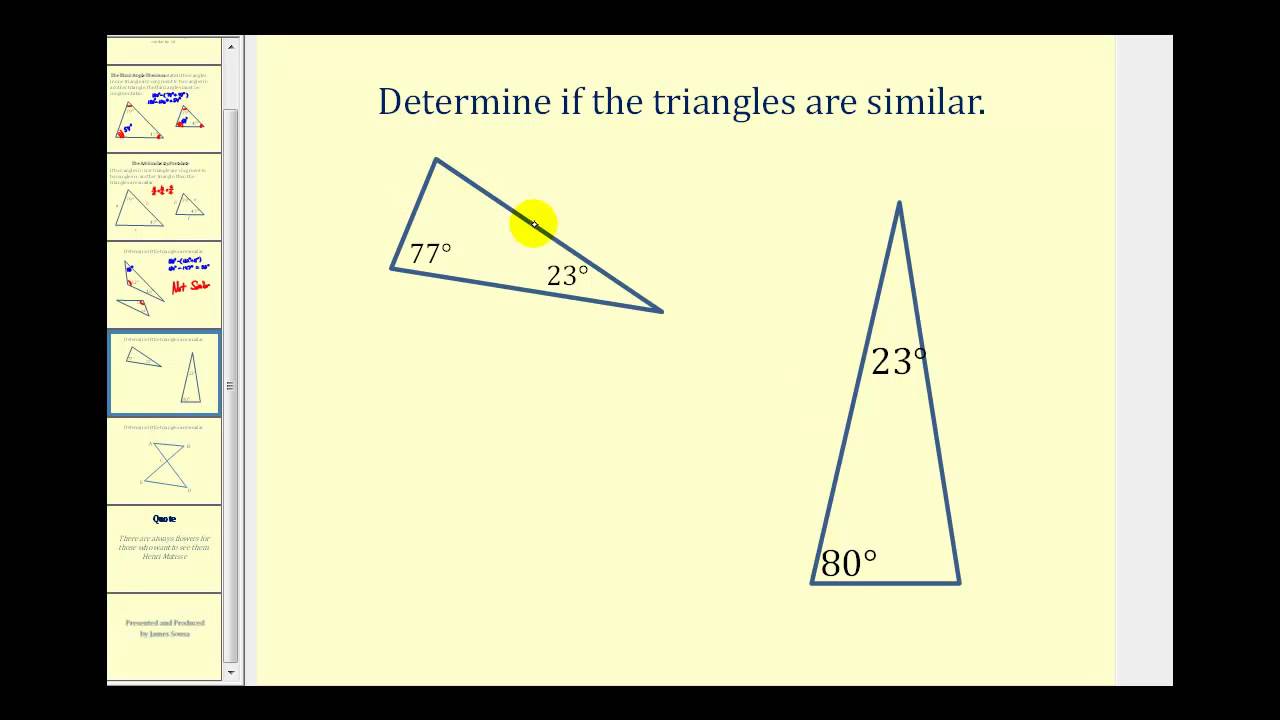
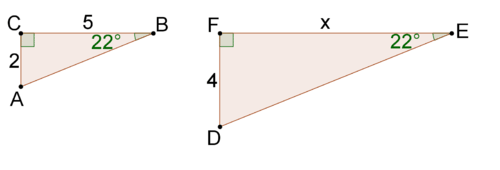
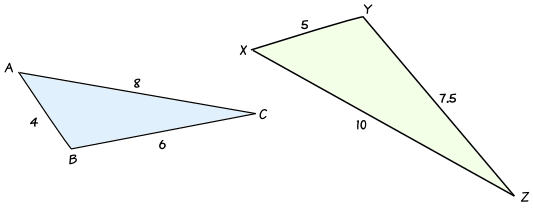
How To Find If Triangles Are Similar


How To Find If Triangles Are Similar
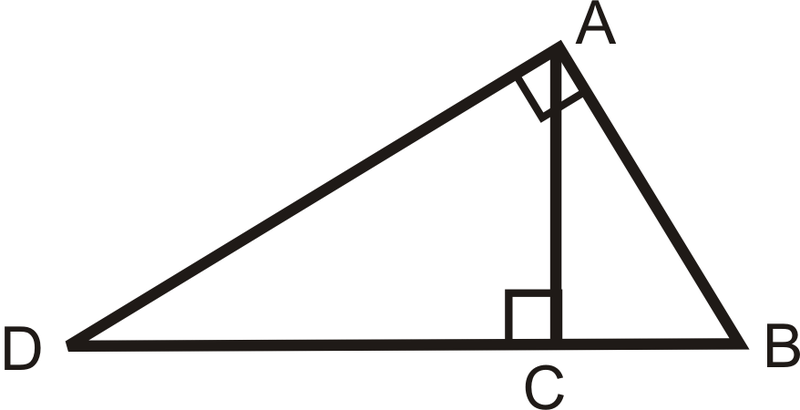


Using Similar Right Triangles Ck 12 Foundation
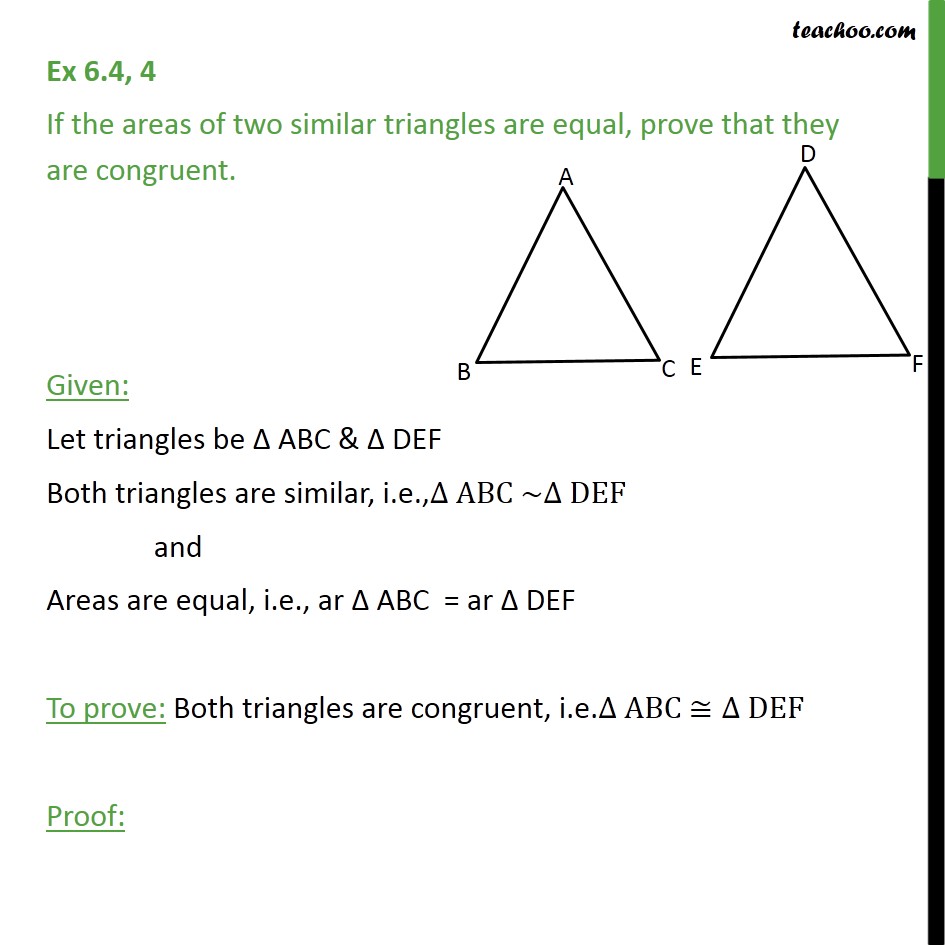


Ex 6 4 4 If Areas Of Two Similar Triangles Are Equal Ex 6 4


Similar Triangles Examples And Problems With Solutions


How To Find If Triangles Are Similar
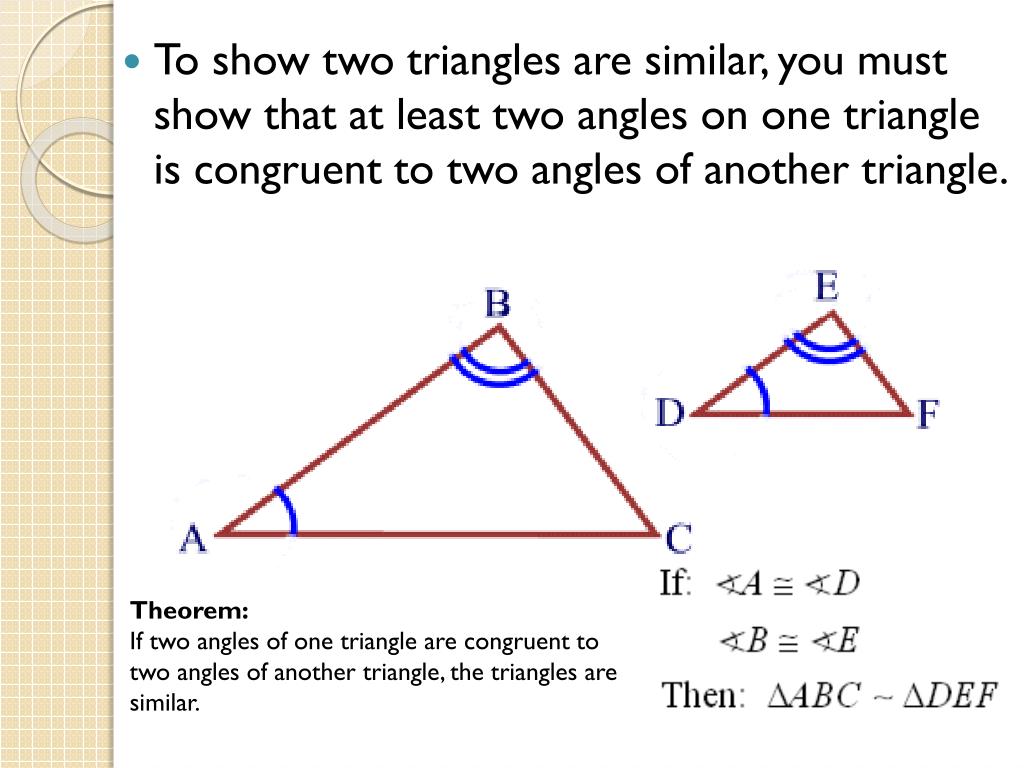


Ppt Aim Proving Triangles Similar Powerpoint Presentation Free Download Id


Proving Similar Triangles Mathbitsnotebook Geo Ccss Math
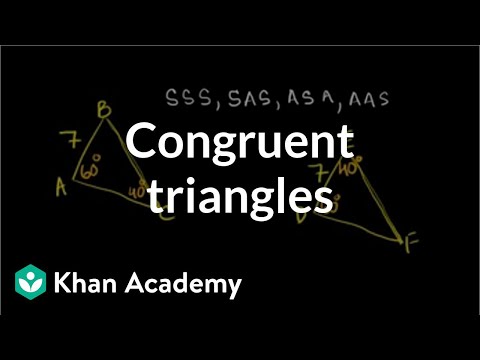


Determining Congruent Triangles Video Khan Academy



Similar Triangles How To Prove Definition Theorems Video
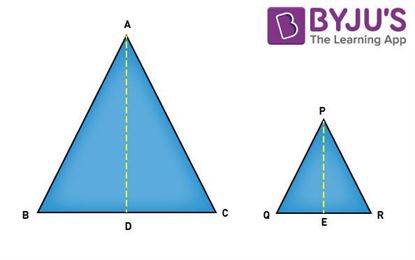


Similar Triangles Formula Theorem Proof Of Sss Sas a Similarity
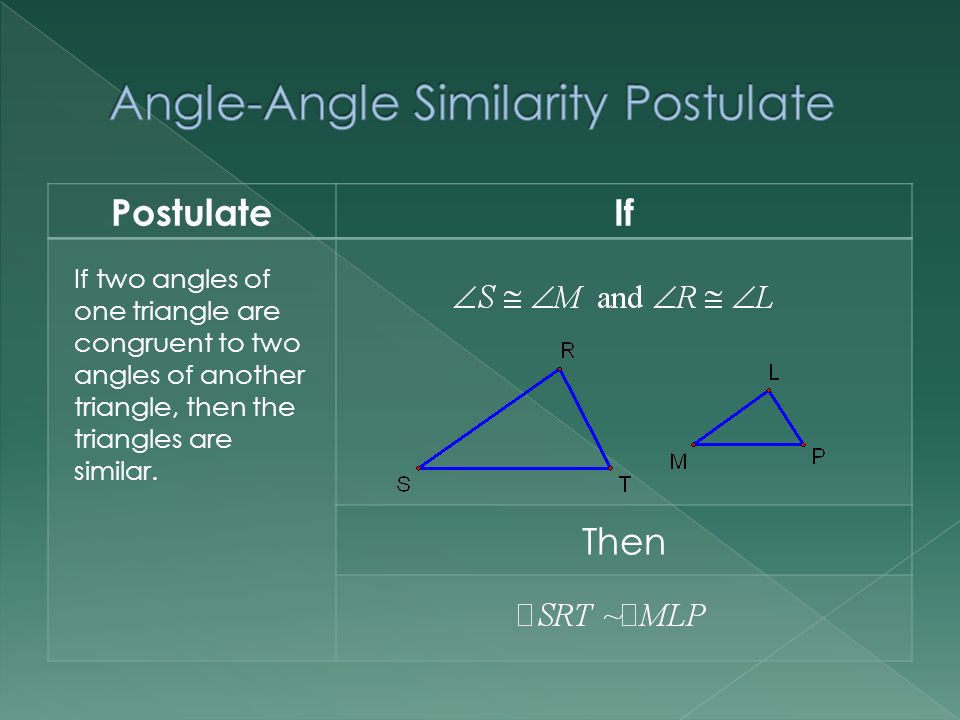


There Are 3 Ways To Show Two Triangles Are Similar To Each Other Those 3 Ways Are 1 Angle Angle Similarity Postulate 2 Side Angle Side Similarity Ppt Download


Similar Triangles Examples And Problems With Solutions



Triangle Similarity Sss Sas a Postulates Proving Similar Triangles Two Column Proofs Youtube


Similar Triangles Perimeters And Areas
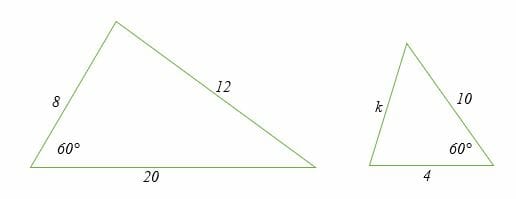


Similar Triangles Explanation Examples



Sas Similarity Read Geometry Ck 12 Foundation
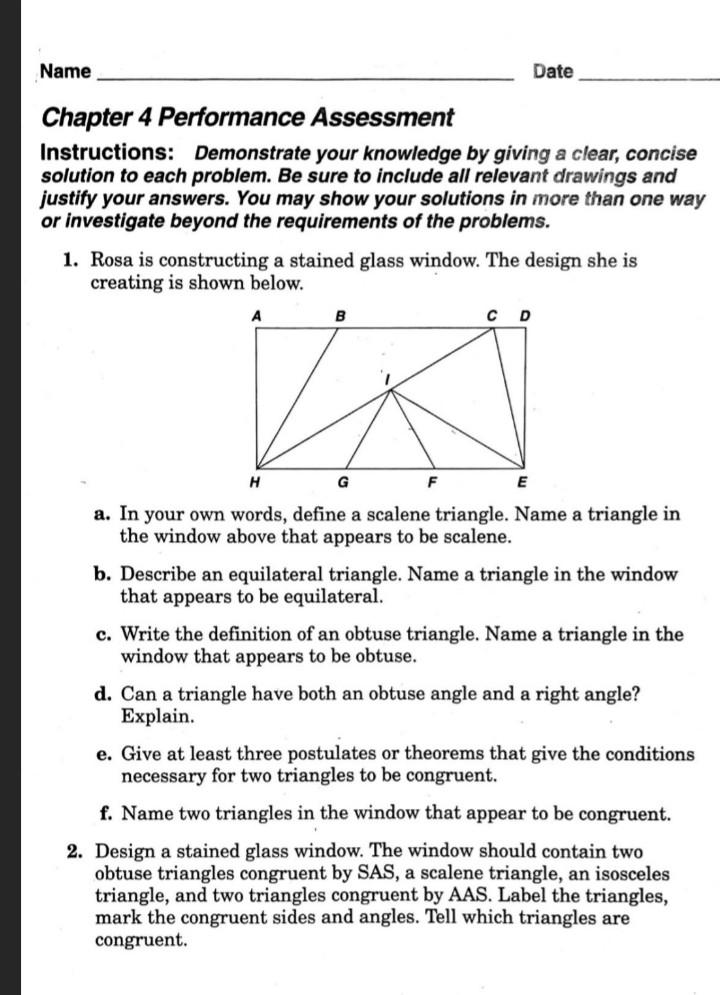


Solved Name Date Chapter 4 Performance Assessment Instruc Chegg Com


Theorems About Similar Triangles


Solved In Exercises 7 10 Show That The Two Tria



Similar Triangles How To Prove Definition Theorems Video


Which Of The Following Can Be Used To Prove That Two Triangles Are Similar Socratic


Triangles



Determine Whether The Two Triangles Are Similar Ppt Download
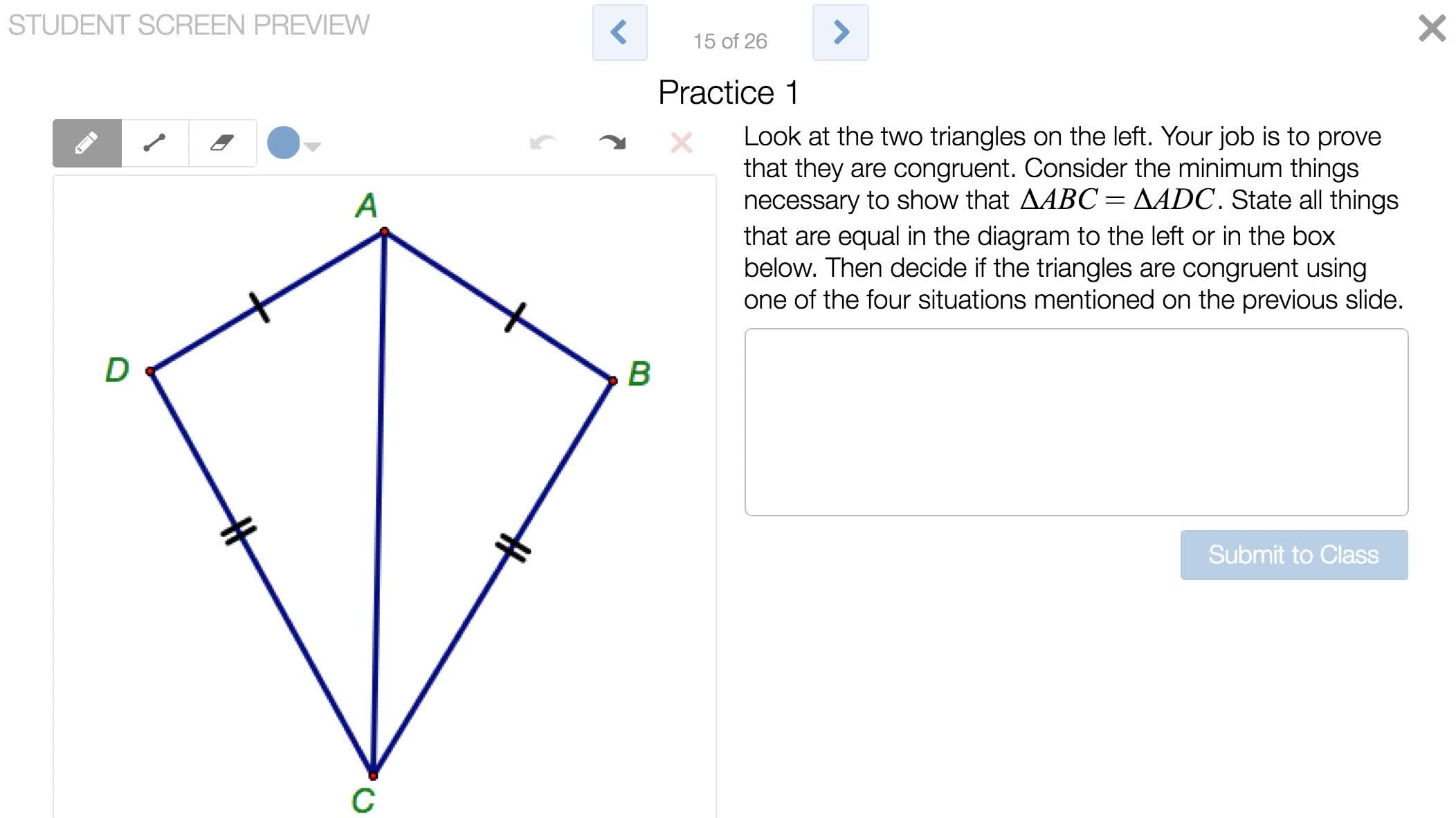


Investigating Congruent Triangles Activity Builder By Desmos
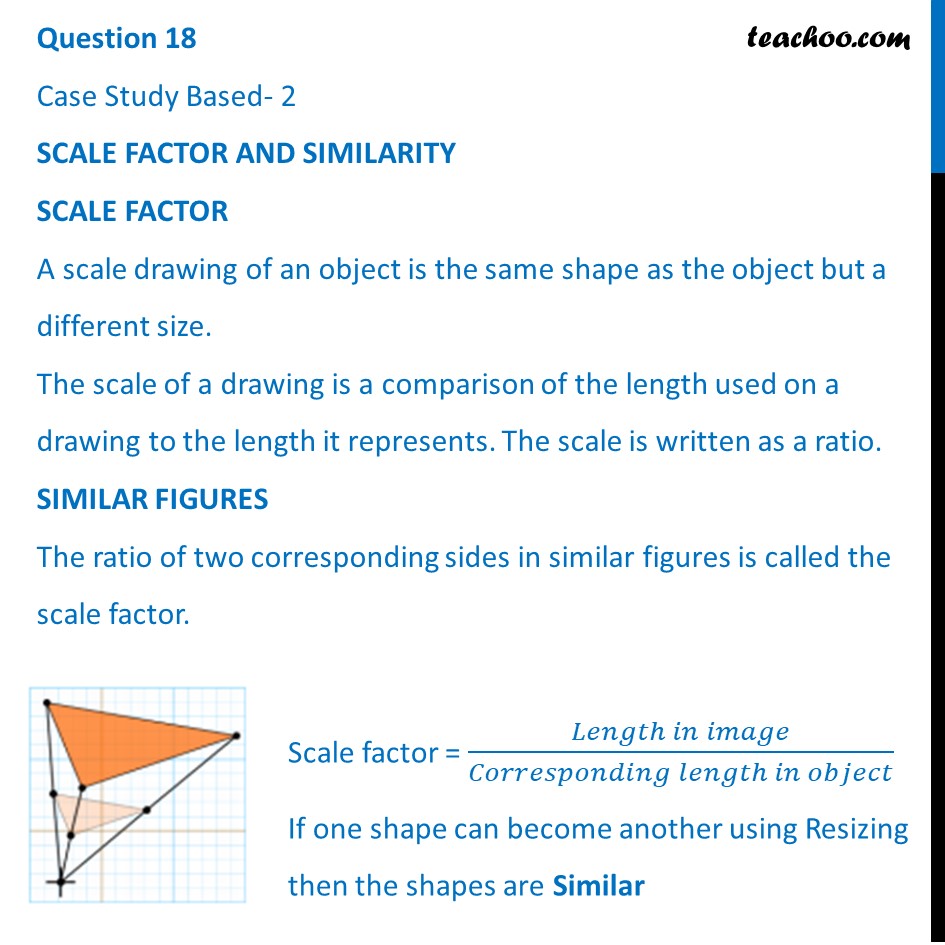


A Scale Drawing Of An Object Is The Same Shape As The Object But A
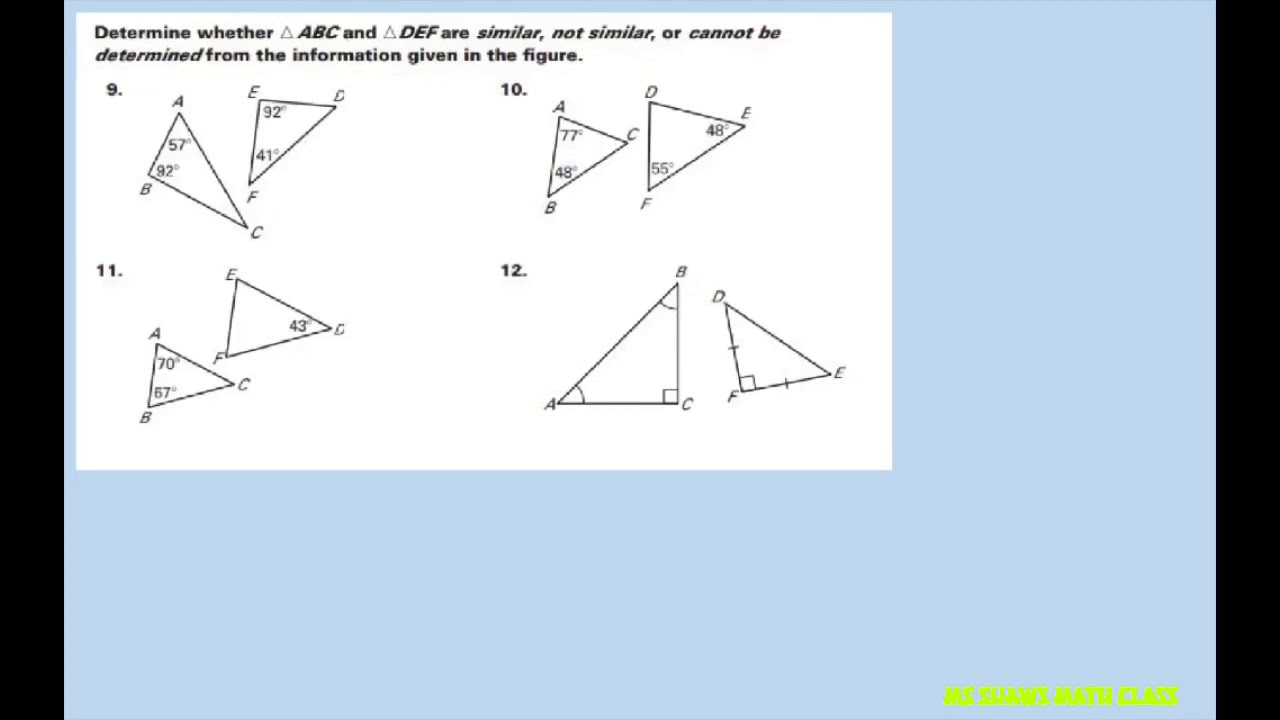


Determine Whether Two Triangles Are Similar Not Similar Or Not Enough Information Youtube
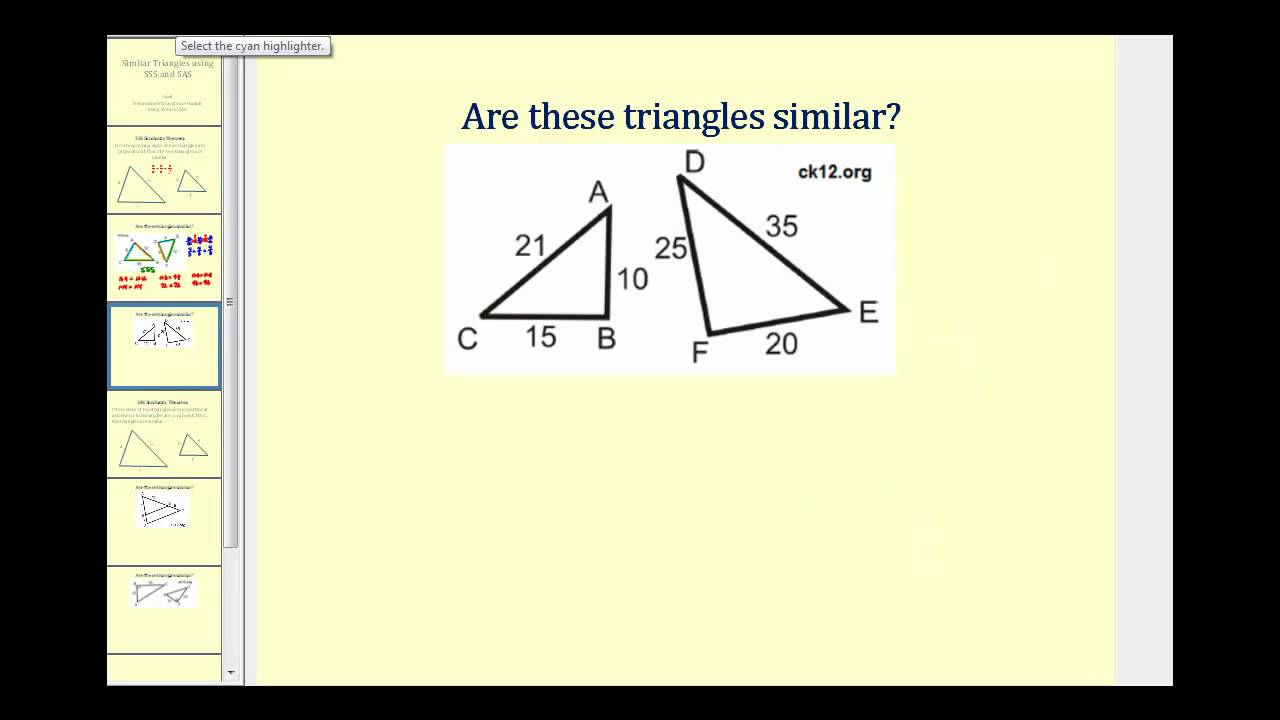


Similar Triangles Solutions Examples Videos



Lesson 7 3 Similar Triangles Ppt Video Online Download


Math Scene Triangles Lesson 1
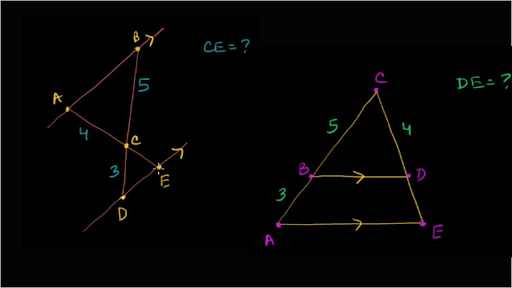


Solving Similar Triangles Video Khan Academy


Proving Similar Triangles Mathbitsnotebook Geo Ccss Math



7 3 Proving Triangles Similar Ppt Download



Congruent Triangles Explanation Examples


Triangles
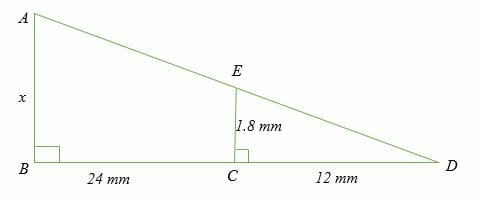


Similar Triangles Explanation Examples
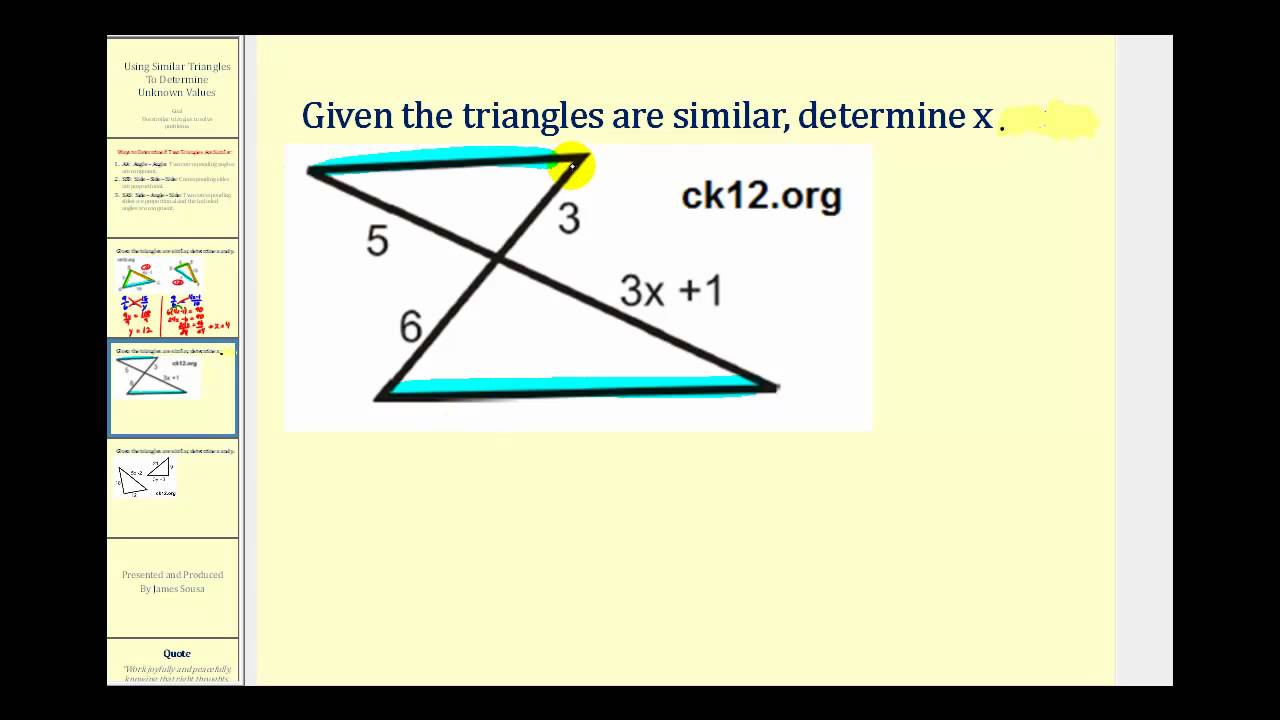


Similar Triangles Solutions Examples Videos
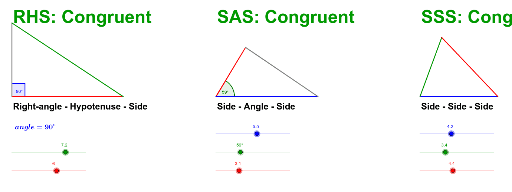


Congruent Triangles Criteria Geogebra
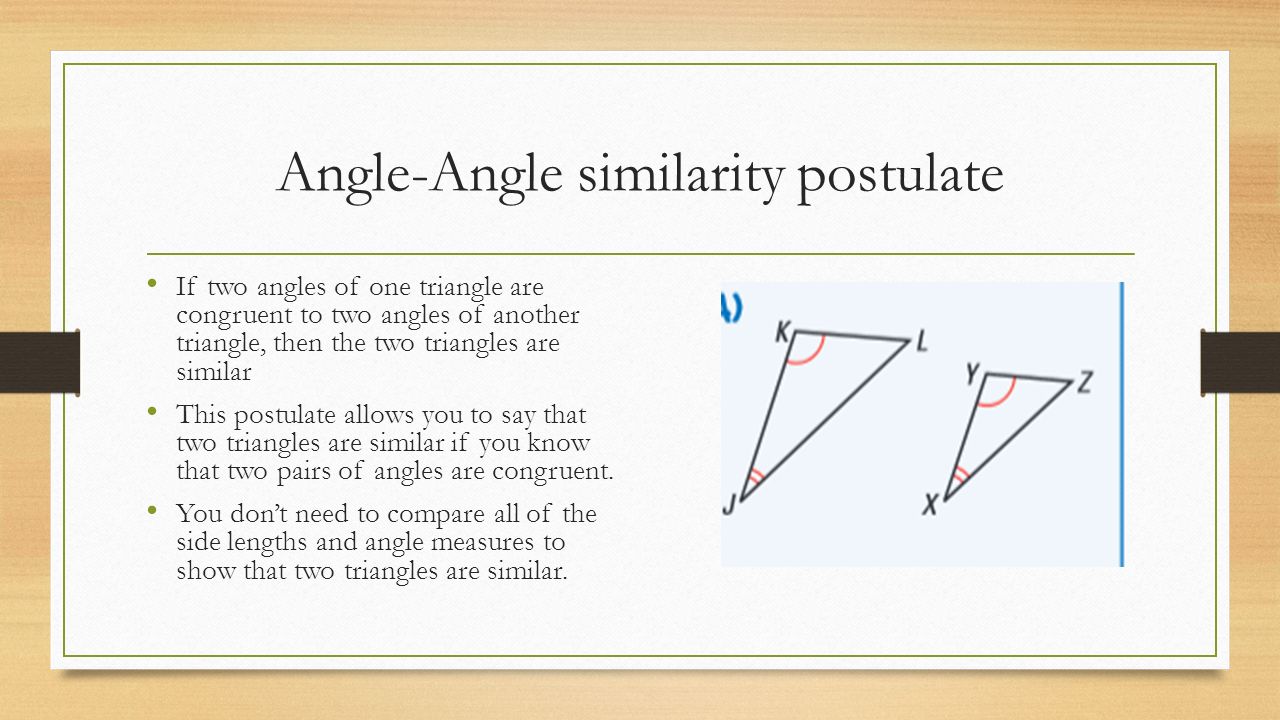


Triangle Similarity Angle Angle Recall Recall The Definitions Of The Following Similar Congruent Also Recall The Properties Of Similarity We Discussed Ppt Download
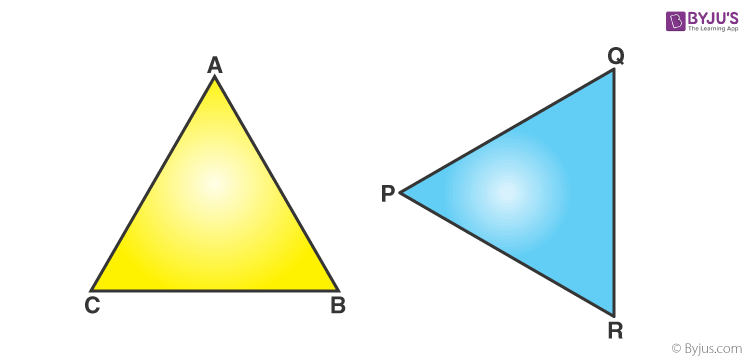


Congruence Of Triangles Conditions Sss Sas Asa And Rhs
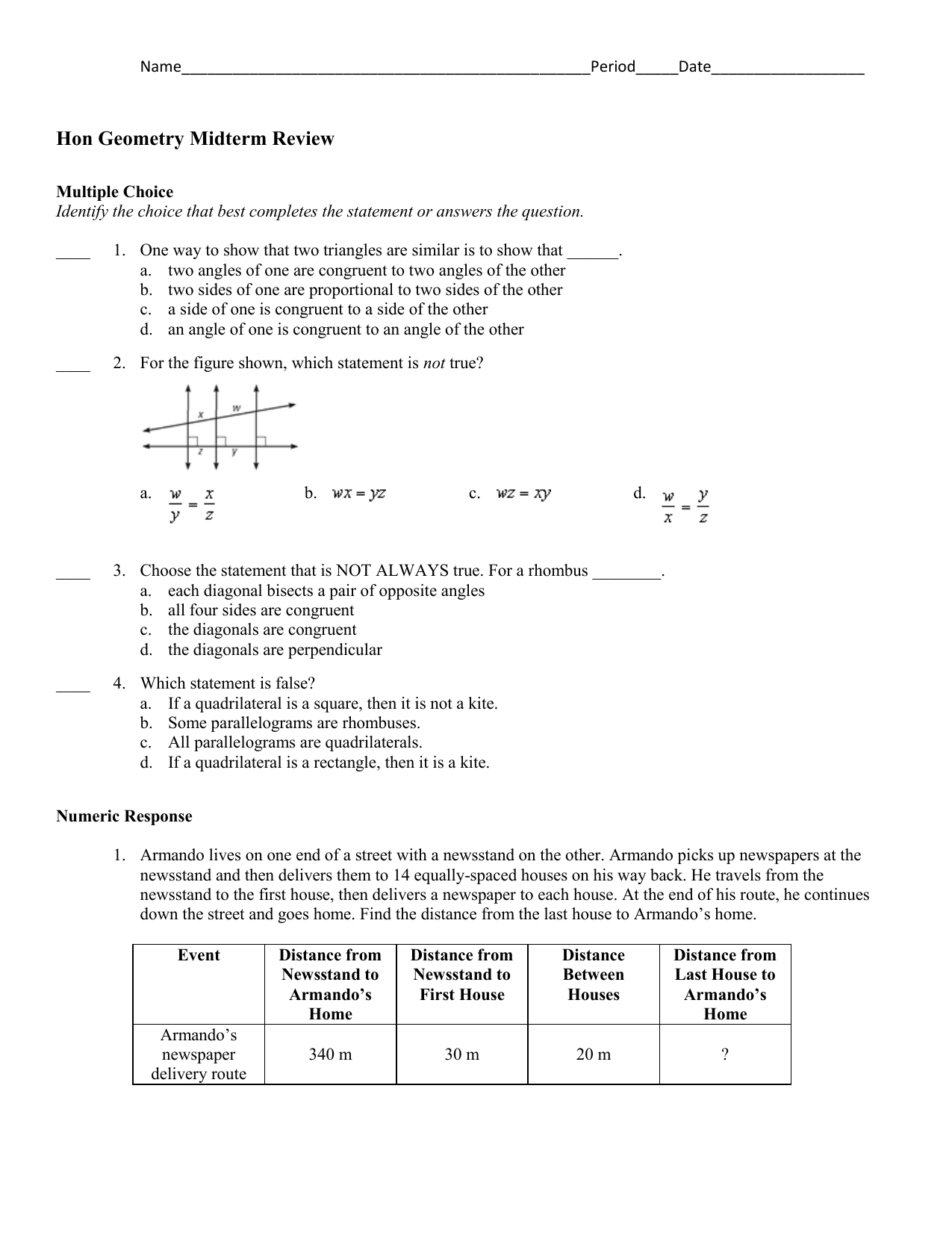


Hon Geometry Midterm Review


Solved Th 4 Given A Convex Quadrilateral Abcd Draw The T Chegg Com



Determine Whether The Two Triangles Are Similar Brainly In
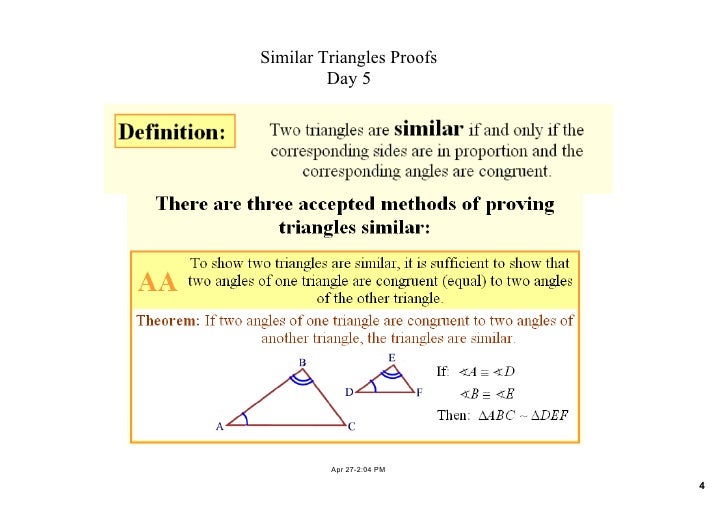


Notes Day 4 5 Prove That Triangles Are Similar



6 4 Prove Triangles Similar By Ppt Download


コメント
コメントを投稿